Der Begriff Prozent
Sicherlich hast du es schon einmal gesehen das „Prozentzeichen“ (%).
Auf Verkehrsschildern, beim Einkaufen, der Auswertung von Wahlen zum Bundestag und natürlich im LADEBALKEN deines Handys kann man es finden.
Prozent stammt vom lateinischen „per cent“ und bedeutet so viel wie „von Hundert“.
Man schreibt:
1 % = \( \frac{1}{100} = 0,01 \)
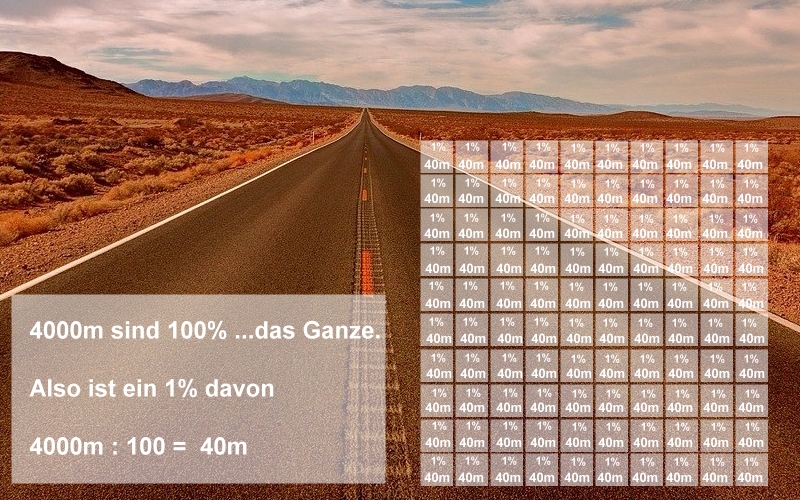
Im Prinzip kann man alle Größen in 100 gleiche Teile aufteilen. Ob 200€ oder 4km oder gar 80.000.000 Einwohner Deutschlands.
Das 100%-Kästchen stellt jedes einzelne Prozent übersichtlich dar.
Das 10er Kästchen ist auch ein guter Helfer. Allerdings eignet es sich nur zur Darstellung der 10%, 20%, 30% usw..
Oder hast du eine Idee, wie es noch einzusetzen wäre?

Auch im 20er Kästchen können Prozentanteile gut dargestellt werden. Hier zählt jedes Kästchen genau 5% , da die 100% auf 20 Kästchen aufgeteilt werden und 100% : 20 = 5%.

Weitere Varianten…

Übungen dazu …
Hier solltest Du auch das Kürzen und Erweitern von Brüchen beherrschen, um Brüche immer wieder in Hundertstel-Brüche umwandeln zu können, aus denen sich dann die Prozentzahlen ergeben!
![]()